Hedging with Delta

As can be seen in the following chart, the price of shares in National Bank of Canada (NA) recently peaked at $73.22, representing an increase of 21.85% in 110 days, for an annualized rate of return of 72.50%. It is unlikely that this pace of growth can be sustained over an extended period of time. In addition, there are signs that the stock’s growth is slowing, since the RSI 5 indicator (Relative Strength Index, 5 periods) has been trending downward since the beginning of November (in contrast to the stock’s price curve). This is no guarantee that NA is about to drop, but the indicator does suggest that the stock is losing momentum. We could therefore conclude that a more or less major correction may be just around the corner.

Chart 1: Daily changes in the price of NA as at December 16, 2019 ($72.10)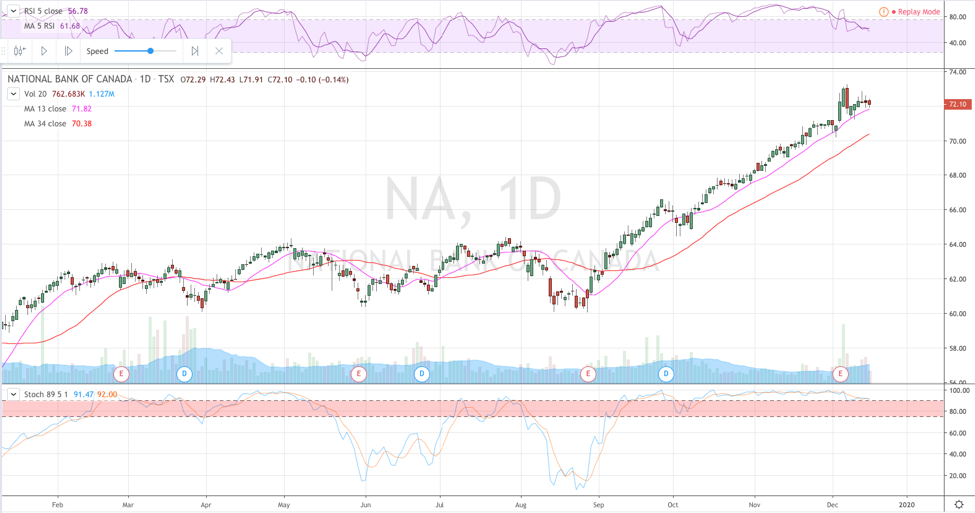
Source: Tradingview.com

Suppose that an investor with a position of 1,000 shares in NA wants to hedge against a short-term decline in the share price. There are two ways to secure this protection: (1) by setting a floor price below which the investor would want to limit losses, or (2) by hedging with delta, which would immunize the investor against a loss due a decline from the current stock price.

The first method involves choosing an exercise price for put options that the investor will buy. For example, the position could be hedged by purchasing 10 put option contracts, for $72 each and maturing on April 17, 2020, at a price of $2.45 per share. If the price of NA falls, the investor’s effective selling price will be $69.55 (the $72 strike price less the $2.45 price of the put). This will result in a loss per share of $2.55 (the current share price of $72.10 less the effective selling price of $69.55), for a total loss of $2,550 on the entire position.

The second method involves determining the right number of contracts, taking into account the delta of the previous put options. Delta is one of the Greek option variables (i.e. measures of the sensitivity of the option price to a change in the value of one of its valuation variables). It measures the change in the option price as a result of a change in the stock price. Values for the Greek variables can be found using the option calculator on the Montréal Exchange’s website (https://m-x.ca/marc_options_calc_en.php). The website also provides option prices.

Table 1: $72 put options expiring April 17, 2020 (121 days remaining)

Source: Montréal Exchange

As can be seen in Table 1, the $72 put options maturing on April 17, 2020 at $2.45 per share have a delta of -0.5644. This means that a $1 decrease in the price of NA will result in the price of the put options increasing by $0.56 per share (-0.5644 x $1.00). So if the share price falls $1, the investor will incur a $1,000 loss on his 1,000 shares, but if the investor buys the right number of put option contracts, this loss will be offset by a $1,000 gain in the price of the put options. The right number of contracts is determined as follows:

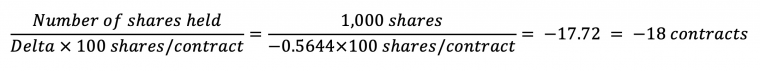
As we can see, by rounding the result of -17.72 contracts to the nearest whole number, we get a figure of -18 contracts. Since this is a negative amount, it represents the number of put options to buy. So the investor will need to buy 18 contracts to be protected against a drop in the price of NA.

Let’s go back to the previous example, in which the price of NA shares fell by $1. We calculated that the investor’s stock position generated a loss of $1,000. Now let’s calculate the gain generated by the 18 put option contracts. With a delta of -0.5644, we expect the price of the put options to increase by $0.56. So, multiplying this gain by 18 contracts of 100 shares each yields a gain of $1,008 (0.56 x 18 x 100). As we can see, this gain fully offsets the loss on the investor’s position caused by the drop in the stock price. This is true if the decline happens quickly, but our scenario may not represent reality, because delta is sensitive to the passage of time and will be changing at the same time as the stock price changes.

Table 2: $72 put options expiring April 17, 2020 (91 days remaining)

Source: Montréal Exchange

Table 2 shows the value of the put option and its delta if the decline occurred over 31 days instead of immediately, leaving only 91 days to expiration. We can see that the stock’s delta would then be only -0.5279, meaning that a $1 decline would generate a gain of $950 (-1 x -0.5279 x 18 x 100) instead of the $1,008 calculated above. This $58 shortfall would be in addition to the decrease in the price of the put options, which are now worth only $1.88 per share, resulting in a loss of $1,026 ([2.45 – 1.88] x 18 x 100) on the put options and making for a total loss of $1,084. However, this is still less than the $2,550 loss we would incur had we used the first method.

This article has presented two hedging strategies: a simpler one that only requires a floor price with a cost that is known in advance, and a more complicated one whose final cost will not be known until later. Hedging with delta allows us to protect our position at the current level, as long as the stock price falls quickly. Otherwise, the loss will be a function of the decay of the options’ time value. The longer that it takes for the stock price to fall, the greater the loss. Therefore, it will be important to re-assess the hedge at the midway point—in this case, when there are only 60 days left until expiration—to determine if it is still required.

Good luck with your trading, and have a good week!

The strategies presented in this blog are for information and training purposes only, and should not be interpreted as recommendations to buy or sell any security. As always, you should ensure that you are comfortable with the proposed scenarios and ready to assume all the risks before implementing an option strategy.